assignment class 10 quadratic equation

CBSE NCERT Solutions

NCERT and CBSE Solutions for free

Class 10 Mathematics Quadratic Equation Assignments

We have provided below free printable Class 10 Mathematics Quadratic Equation Assignments for Download in PDF. The Assignments have been designed based on the latest NCERT Book for Class 10 Mathematics Quadratic Equation . These Assignments for Grade 10 Mathematics Quadratic Equation cover all important topics which can come in your standard 10 tests and examinations. Free printable Assignments for CBSE Class 10 Mathematics Quadratic Equation , school and class assignments, and practice test papers have been designed by our highly experienced class 10 faculty. You can free download CBSE NCERT printable Assignments for Mathematics Quadratic Equation Class 10 with solutions and answers. All Assignments and test sheets have been prepared by expert teachers as per the latest Syllabus in Mathematics Quadratic Equation Class 10. Students can click on the links below and download all Pdf Assignments for Mathematics Quadratic Equation class 10 for free. All latest Kendriya Vidyalaya Class 10 Mathematics Quadratic Equation Assignments with Answers and test papers are given below.

Mathematics Quadratic Equation Class 10 Assignments Pdf Download

We have provided below the biggest collection of free CBSE NCERT KVS Assignments for Class 10 Mathematics Quadratic Equation . Students and teachers can download and save all free Mathematics Quadratic Equation assignments in Pdf for grade 10th. Our expert faculty have covered Class 10 important questions and answers for Mathematics Quadratic Equation as per the latest syllabus for the current academic year. All test papers and question banks for Class 10 Mathematics Quadratic Equation and CBSE Assignments for Mathematics Quadratic Equation Class 10 will be really helpful for standard 10th students to prepare for the class tests and school examinations. Class 10th students can easily free download in Pdf all printable practice worksheets given below.

Topicwise Assignments for Class 10 Mathematics Quadratic Equation Download in Pdf

Advantages of Class 10 Mathematics Quadratic Equation Assignments

As we have the best and largest collection of Mathematics Quadratic Equation assignments for Grade 10, you will be able to easily get full list of solved important questions which can come in your examinations.
Students will be able to go through all important and critical topics given in your CBSE Mathematics Quadratic Equation textbooks for Class 10 .
All Mathematics Quadratic Equation assignments for Class 10 have been designed with answers. Students should solve them yourself and then compare with the solutions provided by us.
Class 10 Students studying in per CBSE, NCERT and KVS schools will be able to free download all Mathematics Quadratic Equation chapter wise worksheets and assignments for free in Pdf
Class 10 Mathematics Quadratic Equation question bank will help to improve subject understanding which will help to get better rank in exams

Frequently Asked Questions by Class 10 Mathematics Quadratic Equation students

At https://www.cbsencertsolutions.com, we have provided the biggest database of free assignments for Mathematics Quadratic Equation Class 10 which you can download in Pdf

We provide here Standard 10 Mathematics Quadratic Equation chapter-wise assignments which can be easily downloaded in Pdf format for free.

You can click on the links above and get assignments for Mathematics Quadratic Equation in Grade 10, all topic-wise question banks with solutions have been provided here. You can click on the links to download in Pdf.

We have provided here topic-wise Mathematics Quadratic Equation Grade 10 question banks, revision notes and questions for all difficult topics, and other study material.

We have provided the best collection of question bank and practice tests for Class 10 for all subjects. You can download them all and use them offline without the internet.

Class 10 Mathematics Arithmetic Progression Assignments

Class 10 Sanskrit Assignments

Class 10 Mathematics Surface Area and Volume Assignments

Search This Blog

Featured posts, math assignment class xi ch-10 | conic sections, math assignment class x ch-4 | quadratic equations.

Math Assignment / Class X / Chapter 4 / Quadratic Equations

Extra questions of chapter 4 class 10 Quadratic Equations with answer and hints to the difficult questions. Important and useful math assignment for the students of class 10

For better results

Students should learn all the basic points of Quadratic Equations
Student should revise N C E R T book thoroughly with examples.
Now revise this assignment. This assignment integrate the knowledge of the students.

ASSIGNMENT FOR 10 STANDARD QUADRATIC EQUATIONS

Theorems on Quadrilaterals Ch-8 Class-IX

Lesson Plan Math Class X (Ch-12) | Surface Area and Volume

Assignment 10 15
Assignment 11 13
Assignment 12 15
Assignment 8 8
Assignment 9 5
Lesson plan 10 15
Lesson Plan 12 14
Lesson Plan 8 10
Maths 10 20
Maths 11 22
Maths 12 18

SUBSCRIBE FOR NEW POSTS

Get new posts by email:.

NCERT Solutions
NCERT Solutions for Class 10
NCERT Solutions for Class 10 Maths
Chapter 4: Quadratic Equations

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations

Ncert solutions class 10 maths chapter 4 – cbse free pdf download.

NCERT Solutions Class 10 Maths Chapter 4 Quadratic Equations contain all the solutions to the problems provided in the Class 10 Maths NCERT textbook for CBSE exam preparations. The questions from every section are framed and solved accurately by the subject experts. NCERT Solutions for Class 10 are detailed and step-by-step guides to all the queries of the students. The exercises present in the chapter should be dealt with utmost sincerity if one wants to score well in the examinations. Maths is a subject that requires a good understanding and a lot of practice. The tips and tricks to solve the problems easily are also provided here. A quadratic equation in the variable x is an equation of the form ax 2 + bx + c = 0, where a, b, c are real numbers, a ≠ 0. That is, ax 2 + bx + c = 0, a ≠ 0 is called the standard form of a quadratic equation.

Download Exclusively Curated Chapter Notes for Class 10 Maths Chapter – 4 Quadratic Equations

Download most important questions for class 10 maths chapter – 4 quadratic equations.

Quadratic equations arise in several situations around us. Hence, students should give special attention to learning the concepts related to this chapter of the latest CBSE Syllabus for 2023-24 thoroughly to excel in Class 10 Maths examinations. NCERT Solutions help the students in learning these concepts as well as in evaluating themselves. Practising these solutions repeatedly is bound to help the students in overcoming their shortcomings. Maths has either a correct answer or a wrong one. Therefore, it is imperative to concentrate while solving the questions to score full marks.

Chapter 1 Real Numbers
Chapter 2 Polynomials
Chapter 3 Pair of Linear Equations in Two Variables
Chapter 4 Quadratic Equations
Chapter 5 Arithmetic Progressions
Chapter 6 Triangles
Chapter 7 Coordinate Geometry
Chapter 8 Introduction to Trigonometry
Chapter 9 Some Applications of Trigonometry
Chapter 10 Circles
Chapter 11 Constructions
Chapter 12 Areas Related to Circles
Chapter 13 Surface Areas and Volumes
Chapter 14 Statistics
Chapter 15 Probability
Exercise 4.1
Exercise 4.2
Exercise 4.3
Exercise 4.4

NCERT Solutions Class 10 Maths Chapter 4 – Quadratic Equations

carouselExampleControls112

NCERT Solutions for Class 10 Maths Chapter 4 Quadratic Equations

Previous Next

Access answers to NCERT Class 10 Maths Chapter 4 – Quadratic Equations

Exercise 4.1 page: 73.

1. Check whether the following are quadratic equations:

(i) (x + 1) 2 = 2(x – 3)

(ii) x 2 – 2x = (–2) (3 – x)

(iii) (x – 2)(x + 1) = (x – 1)(x + 3)

(iv) (x – 3)(2x +1) = x(x + 5)

(v) (2x – 1)(x – 3) = (x + 5)(x – 1)

(vi) x 2 + 3x + 1 = (x – 2) 2

(vii) (x + 2) 3 = 2x (x 2 – 1)

(viii) x 3 – 4x 2 – x + 1 = (x – 2) 3

(x + 1) 2 = 2(x – 3)

By using the formula for (a+b) 2 = a 2 +2ab+b 2

⇒ x 2 + 2x + 1 = 2x – 6

⇒ x 2 + 7 = 0

The above equation is in the form of ax 2 + bx + c = 0

Therefore, the given equation is a quadratic equation.

(ii) Given, x 2 – 2x = (–2) (3 – x)

⇒ x 2 – 2x = -6 + 2x

⇒ x 2 – 4x + 6 = 0

(iii) Given, (x – 2)(x + 1) = (x – 1)(x + 3)

By multiplication,

⇒ x 2 – x – 2 = x 2 + 2x – 3

⇒ 3x – 1 = 0

The above equation is not in the form of ax 2 + bx + c = 0

Therefore, the given equation is not a quadratic equation.

(iv) Given, (x – 3)(2x +1) = x(x + 5)

⇒ 2x 2 – 5x – 3 = x 2 + 5x

⇒ x 2 – 10x – 3 = 0

(v) Given, (2x – 1)(x – 3) = (x + 5)(x – 1)

⇒ 2x 2 – 7x + 3 = x 2 + 4x – 5

⇒ x 2 – 11x + 8 = 0

The above equation is in the form of ax 2 + bx + c = 0.

(vi) Given, x 2 + 3x + 1 = (x – 2) 2

By using the formula for (a-b) 2 =a 2 -2ab+b 2

⇒ x 2 + 3x + 1 = x 2 + 4 – 4x

⇒ 7x – 3 = 0

(vii) Given, (x + 2) 3 = 2x(x 2 – 1)

By using the formula for (a+b) 3 = a 3 +b 3 +3ab(a+b)

⇒ x 3 + 8 + x 2 + 12x = 2x 3 – 2x

⇒ x 3 + 14x – 6x 2 – 8 = 0

(viii) Given, x 3 – 4x 2 – x + 1 = (x – 2) 3

By using the formula for (a-b) 3 = a 3 -b 3 -3ab(a-b)

⇒ x 3 – 4x 2 – x + 1 = x 3 – 8 – 6x 2 + 12x

⇒ 2x 2 – 13x + 9 = 0

2. Represent the following situations in the form of quadratic equations:

(i) The area of a rectangular plot is 528 m 2 . The length of the plot (in metres) is one more than twice its breadth. We need to find the length and breadth of the plot.

(ii) The product of two consecutive positive integers is 306. We need to find the integers.

(iii) Rohan’s mother is 26 years older than him. The product of their ages (in years) 3 years from now will be 360. We would like to find Rohan’s present age.

(iv) A train travels a distance of 480 km at a uniform speed. If the speed had been 8 km/h less, then it would have taken

(i) Let us consider,

The breadth of the rectangular plot = x m

Thus, the length of the plot = (2x + 1) m

As we know,

Area of rectangle = length × breadth = 528 m 2

Putting the value of the length and breadth of the plot in the formula, we get,

(2x + 1) × x = 528

⇒ 2x 2 + x =528

⇒ 2x 2 + x – 528 = 0

Therefore, the length and breadth of the plot satisfy the quadratic equation, 2x 2 + x – 528 = 0, which is the required representation of the problem mathematically.

(ii) Let us consider,

The first integer number = x

Thus, the next consecutive positive integer will be = x + 1

Product of two consecutive integers = x × (x +1) = 306

⇒ x 2 + x = 306

⇒ x 2 + x – 306 = 0

Therefore, the two integers x and x+1 satisfy the quadratic equation, x 2 + x – 306 = 0, which is the required representation of the problem mathematically.

(iii) Let us consider,

Age of Rohan’s = x years

Therefore, as per the given question,

Rohan’s mother’s age = x + 26

After 3 years,

Age of Rohan’s = x + 3

Age of Rohan’s mother will be = x + 26 + 3 = x + 29

The product of their ages after 3 years will be equal to 360, such that

(x + 3)(x + 29) = 360

⇒ x 2 + 29x + 3x + 87 = 360

⇒ x 2 + 32x + 87 – 360 = 0

⇒ x 2 + 32x – 273 = 0

Therefore, the age of Rohan and his mother satisfies the quadratic equation, x 2 + 32x – 273 = 0, which is the required representation of the problem mathematically.

(iv) Let us consider,

The speed of the train = x km/h

Time taken to travel 480 km = 480/x km/hr

As per second condition, the speed of train = ( x – 8) km/h

Also given, the train will take 3 hours to cover the same distance.

Therefore, time taken to travel 480 km = (480/x)+3 km/h

Speed × Time = Distance

( x – 8)(480/ x )+ 3 = 480

⇒ 480 + 3 x – 3840/ x – 24 = 480

⇒ 3 x – 3840/ x = 24

⇒ x 2 – 8 x – 1280 = 0

Therefore, the speed of the train satisfies the quadratic equation, x 2 – 8 x – 1280 = 0, which is the required representation of the problem mathematically.

Exercise 4.2 Page: 76

1. Find the roots of the following quadratic equations by factorisation:

(i) x 2 – 3x – 10 = 0 (ii) 2x 2 + x – 6 = 0 (iii) √2 x 2 + 7x + 5√2 = 0 (iv) 2x 2 – x +1/8 = 0 (v) 100x 2 – 20x + 1 = 0

(i) Given, x 2 – 3 x – 10 =0

Taking L.H.S.,

=> x 2 – 5 x + 2 x – 10

=> x ( x – 5) + 2( x – 5)

=>( x – 5)( x + 2)

The roots of this equation, x 2 – 3 x – 10 = 0 are the values of x for which ( x – 5)( x + 2) = 0

Therefore, x – 5 = 0 or x + 2 = 0

=> x = 5 or x = -2

(ii) Given, 2 x 2 + x – 6 = 0

=> 2 x 2 + 4 x – 3 x – 6

=> 2 x ( x + 2) – 3( x + 2)

=> ( x + 2)(2 x – 3)

The roots of this equation, 2 x 2 + x – 6=0 are the values of x for which ( x x + 2)(2 x – 3) = 0

Therefore, x + 2 = 0 or 2 x – 3 = 0

=> x = -2 or x = 3/2

(iii) √2 x 2 + 7 x + 5√2=0

=> √2 x 2 + 5 x + 2 x + 5√2

=> x (√2 x + 5) + √2(√2 x + 5)= (√2 x + 5)( x + √2)

The roots of this equation, √2 x 2 + 7 x + 5√2=0 are the values of x for which (√2 x + 5)( x + √2) = 0

Therefore, √2 x + 5 = 0 or x + √2 = 0

=> x = -5/√2 or x = -√2

(iv) 2 x 2 – x +1/8 = 0

=1/8 (16 x 2 – 8 x + 1)

= 1/8 (16 x 2 – 4 x -4 x + 1)

= 1/8 (4 x (4 x – 1) -1(4 x – 1))

= 1/8 (4 x – 1) 2

The roots of this equation, 2 x 2 – x + 1/8 = 0, are the values of x for which (4 x – 1) 2 = 0

Therefore, (4 x – 1) = 0 or (4 x – 1) = 0

⇒ x = 1/4 or x = 1/4

(v) Given, 100x 2 – 20x + 1=0

= 100x 2 – 10x – 10x + 1

= 10x(10x – 1) -1(10x – 1)

= (10x – 1) 2

The roots of this equation, 100x 2 – 20x + 1=0, are the values of x for which (10x – 1) 2 = 0

∴ (10x – 1) = 0 or (10x – 1) = 0

⇒x = 1/10 or x = 1/10

2. Solve the problems given in Example 1.

Represent the following situations mathematically:

(i) John and Jivanti together have 45 marbles. Both of them lost 5 marbles each, and the product of the number of marbles they now have is 124. We would like to find out how many marbles they had to start with.

(ii) A cottage industry produces a certain number of toys in a day. The cost of production of each toy (in rupees) was found to be 55 minus the number of toys produced in a day. On a particular day, the total cost of production was Rs. 750. We would like to find out the number of toys produced on that day.

(i) Let us say the number of marbles John has = x

Therefore, the number of marble Jivanti has = 45 – x

After losing 5 marbles each,

Number of marbles John has = x – 5

Number of marble Jivanti has = 45 – x – 5 = 40 – x

Given that the product of their marbles is 124.

∴ ( x – 5)(40 – x ) = 124

⇒ x 2 – 45 x + 324 = 0

⇒ x 2 – 36 x – 9 x + 324 = 0

⇒ x ( x – 36) -9( x – 36) = 0

⇒ ( x – 36)( x – 9) = 0

Thus, we can say,

x – 36 = 0 or x – 9 = 0

⇒ x = 36 or x = 9

If John’s marbles = 36

Then, Jivanti’s marbles = 45 – 36 = 9

And if John’s marbles = 9

Then, Jivanti’s marbles = 45 – 9 = 36

(ii) Let us say the number of toys produced in a day is x .

Therefore, cost of production of each toy = Rs(55 – x )

Given the total cost of production of the toys = Rs 750

∴ x (55 – x ) = 750

⇒ x 2 – 55 x + 750 = 0

⇒ x 2 – 25 x – 30 x + 750 = 0

⇒ x ( x – 25) -30( x – 25) = 0

⇒ ( x – 25)( x – 30) = 0

Thus, either x -25 = 0 or x – 30 = 0

⇒ x = 25 or x = 30

Hence, the number of toys produced in a day will be either 25 or 30.

3. Find two numbers whose sum is 27 and product is 182.

Let us say the first number is x, and the second number is 27 – x.

Therefore, the product of two numbers

x(27 – x) = 182

⇒ x 2 – 27x – 182 = 0

⇒ x 2 – 13x – 14x + 182 = 0

⇒ x(x – 13) -14(x – 13) = 0

⇒ (x – 13)(x -14) = 0

Thus, either, x = -13 = 0 or x – 14 = 0

⇒ x = 13 or x = 14

Therefore, if first number = 13, then second number = 27 – 13 = 14

And if first number = 14, then second number = 27 – 14 = 13

Hence, the numbers are 13 and 14.

4. Find two consecutive positive integers, the sum of whose squares is 365.

Let us say the two consecutive positive integers are x and x + 1.

Therefore, as per the given questions,

x 2 + ( x + 1) 2 = 365

⇒ x 2 + x 2 + 1 + 2 x = 365

⇒ 2 x 2 + 2x – 364 = 0

⇒ x 2 + x – 182 = 0

⇒ x 2 + 14 x – 13 x – 182 = 0

⇒ x ( x + 14) -13( x + 14) = 0

⇒ ( x + 14)( x – 13) = 0

Thus, either, x + 14 = 0 or x – 13 = 0,

⇒ x = – 14 or x = 13

Since the integers are positive, x can be 13 only.

∴ x + 1 = 13 + 1 = 14

Therefore, two consecutive positive integers will be 13 and 14.

5. The altitude of a right triangle is 7 cm less than its base. If the hypotenuse is 13 cm, find the other two sides.

Let us say the base of the right triangle is x cm.

Given, the altitude of right triangle = (x – 7) cm

From Pythagoras’ theorem, we know,

Base 2 + Altitude 2 = Hypotenuse 2

∴ x 2 + (x – 7) 2 = 13 2

⇒ x 2 + x 2 + 49 – 14x = 169

⇒ 2x 2 – 14x – 120 = 0

⇒ x 2 – 7x – 60 = 0

⇒ x 2 – 12x + 5x – 60 = 0

⇒ x(x – 12) + 5(x – 12) = 0

⇒ (x – 12)(x + 5) = 0

Thus, either x – 12 = 0 or x + 5 = 0,

⇒ x = 12 or x = – 5

Since sides cannot be negative, x can only be 12.

Therefore, the base of the given triangle is 12 cm, and the altitude of this triangle will be (12 – 7) cm = 5 cm.

6. A cottage industry produces a certain number of pottery articles in a day. It was observed on a particular day that the cost of production of each article (in rupees) was 3 more than twice the number of articles produced on that day. If the total cost of production on that day was Rs.90, find the number of articles produced and the cost of each article.

Let us say the number of articles produced is x .

Therefore, cost of production of each article = Rs (2 x + 3)

Given the total cost of production is Rs.90

∴ x (2 x + 3) = 90

⇒ 2 x 2 + 3 x – 90 = 0

⇒ 2 x 2 + 15 x -12 x – 90 = 0

⇒ x (2 x + 15) -6(2 x + 15) = 0

⇒ (2 x + 15)( x – 6) = 0

Thus, either 2 x + 15 = 0 or x – 6 = 0

⇒ x = -15/2 or x = 6

As the number of articles produced can only be a positive integer, x can only be 6.

Hence, the number of articles produced = 6

Cost of each article = 2 × 6 + 3 = Rs 15

Exercise 4.3 Page: 87

1. Find the roots of the following quadratic equations, if they exist, by the method of completing the square:

(i) 2 x 2 – 7 x +3 = 0

(ii) 2 x 2 + x – 4 = 0 (iii) 4 x 2 + 4√3 x + 3 = 0

(iv) 2 x 2 + x + 4 = 0

(i) 2 x 2 – 7 x + 3 = 0

⇒ 2 x 2 – 7 x = – 3

Dividing by 2 on both sides, we get

⇒ x 2 -7x/2 = -3/2

⇒ x 2 -2 × x ×7/4 = -3/2

On adding (7/4) 2 to both sides of the equation, we get

⇒ (x) 2 -2×x×7/4 +(7/4) 2 = (7/4) 2 -3/2

⇒ (x-7/4) 2 = (49/16) – (3/2)

⇒(x-7/4) 2 = 25/16

⇒(x-7/4) 2 = ±5/4

⇒ x = 7/4 ± 5/4

⇒ x = 7/4 + 5/4 or x = 7/4 – 5/4

⇒ x = 12/4 or x = 2/4

⇒ x = 3 or x = 1/2

(ii) 2x 2 + x – 4 = 0

⇒ 2x 2 + x = 4

Dividing both sides of the equation by 2, we get

⇒ x 2 +x/2 = 2

Now on adding (1/4) 2 to both sides of the equation, we get,

⇒ (x) 2 + 2 × x × 1/4 + (1/4) 2 = 2 + (1/4) 2

⇒ (x + 1/4) 2 = 33/16

⇒ x + 1/4 = ± √33/4

⇒ x = ± √33/4 – 1/4

⇒ x = (± √33-1)/4

Therefore, either x = (√33-1)/4 or x = (-√33-1)/4

(iii) 4x 2 + 4√3x + 3 = 0

Converting the equation into a 2 +2ab+b 2 form, we get,

⇒ (2x) 2 + 2 × 2x × √3 + (√3) 2 = 0

⇒ (2x + √3) 2 = 0

⇒ (2x + √3) = 0 and (2x + √3) = 0

Therefore, either x = -√3/2 or x = -√3/2

(iv) 2x 2 + x + 4 = 0

⇒ 2x 2 + x = -4

⇒ x 2 + 1/2x = 2

⇒ x 2 + 2 × x × 1/4 = -2

By adding (1/4) 2 to both sides of the equation, we get

⇒ (x) 2 + 2 × x × 1/4 + (1/4) 2 = (1/4) 2 – 2

⇒ (x + 1/4) 2 = 1/16 – 2

⇒ (x + 1/4) 2 = -31/16

As we know, the square of numbers cannot be negative.

Therefore, there is no real root for the given equation, 2x 2 + x + 4 = 0

2. Find the roots of the quadratic equations given in Q.1 above by applying the quadratic formula.

(i) 2x 2 – 7x + 3 = 0

On comparing the given equation with ax 2 + bx + c = 0, we get,

a = 2, b = -7 and c = 3

By using the quadratic formula, we get,

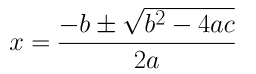
⇒ x = (7±√(49 – 24))/4

⇒ x = (7±√25)/4

⇒ x = (7±5)/4

⇒ x = (7+5)/4 or x = (7-5)/4

⇒ x = 12/4 or 2/4

∴ x = 3 or 1/2

(ii) 2x 2 + x – 4 = 0

a = 2, b = 1 and c = -4

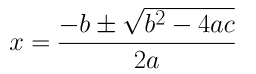
⇒x = (-1±√1+32)/4

⇒x = (-1±√33)/4

∴ x = (-1+√33)/4 or x = (-1-√33)/4

(iii) 4x 2 + 4√3x + 3 = 0

On comparing the given equation with ax 2 + bx + c = 0, we get

a = 4, b = 4√3 and c = 3

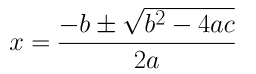
⇒ x = (-4√3±√48-48)/8

⇒ x = (-4√3±0)/8

∴ x = -√3/2 or x = -√3/2

(iv) 2x 2 + x + 4 = 0

a = 2, b = 1 and c = 4

By using the quadratic formula, we get

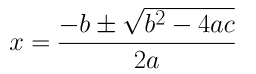
⇒ x = (-1±√1-32)/4

⇒ x = (-1±√-31)/4

As we know, the square of a number can never be negative. Therefore, there is no real solution for the given equation.

3. Find the roots of the following equations:

(i) x-1/x = 3, x ≠ 0 (ii) 1/x+4 – 1/x-7 = 11/30, x = -4, 7

(i) x-1/x = 3

⇒ x 2 – 3x -1 = 0

a = 1, b = -3 and c = -1

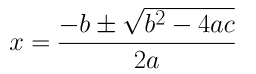
⇒ x = (3±√9+4)/2

⇒ x = (3±√13)/2

∴ x = (3+√13)/2 or x = (3-√13)/2

(ii) 1/x+4 – 1/x-7 = 11/30 ⇒ x-7-x-4/(x+4)(x-7) = 11/30

⇒ -11/(x+4)(x-7) = 11/30

⇒ (x+4)(x-7) = -30

⇒ x 2 – 3x – 28 = 30

⇒ x 2 – 3x + 2 = 0

We can solve this equation by factorisation method now.

⇒ x 2 – 2x – x + 2 = 0

⇒ x(x – 2) – 1(x – 2) = 0

⇒ (x – 2)(x – 1) = 0

⇒ x = 1 or 2

4. The sum of the reciprocals of Rehman’s age (in years) 3 years ago and 5 years from now is 1/3. Find his present age.

Let us say the present age of Rahman is x years.

Three years ago, Rehman’s age was ( x – 3) years.

Five years after, his age will be ( x + 5) years.

Given the sum of the reciprocals of Rehman’s ages 3 years ago and after 5 years is equal to 1/3.

∴ 1/ x -3 + 1/ x -5 = 1/3

(x +5+ x -3)/( x -3)( x +5) = 1/3

(2 x +2)/( x -3)( x +5) = 1/3

⇒ 3(2 x + 2) = ( x -3)( x +5)

⇒ 6 x + 6 = x 2 + 2 x – 15

⇒ x 2 – 4 x – 21 = 0

⇒ x 2 – 7 x + 3 x – 21 = 0

⇒ x ( x – 7) + 3( x – 7) = 0

⇒ ( x – 7)( x + 3) = 0

⇒ x = 7, -3

As we know, age cannot be negative.

Therefore, Rahman’s present age is 7 years.

5. In a class test, the sum of Shefali’s marks in Mathematics and English is 30. Had she got 2 marks more in Mathematics and 3 marks less in English, the product of their marks would have been 210. Find her marks in the two subjects.

Let us say the marks of Shefali in Maths be x.

Then, the marks in English will be 30 – x.

As per the given question,

(x + 2)(30 – x – 3) = 210

(x + 2)(27 – x) = 210

⇒ -x 2 + 25x + 54 = 210

⇒ x 2 – 25x + 156 = 0

⇒ x 2 – 12x – 13x + 156 = 0

⇒ x(x – 12) -13(x – 12) = 0

⇒ (x – 12)(x – 13) = 0

⇒ x = 12, 13

Therefore, if the marks in Maths are 12, then marks in English will be 30 – 12 = 18, and if the marks in Maths are 13, then marks in English will be 30 – 13 = 17 .

6. The diagonal of a rectangular field is 60 metres more than the shorter side. If the longer side is 30 metres more than the shorter side, find the sides of the field.

Let us say the shorter side of the rectangle is x m.

Then, larger side of the rectangle = ( x + 30) m

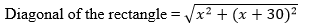
As given, the length of the diagonal is = x + 30 m

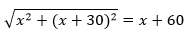
⇒ x 2 + ( x + 30) 2 = ( x + 60) 2

⇒ x 2 + x 2 + 900 + 60 x = x 2 + 3600 + 120 x

⇒ x 2 – 60 x – 2700 = 0

⇒ x 2 – 90 x + 30 x – 2700 = 0

⇒ x ( x – 90) + 30( x -90) = 0

⇒ ( x – 90)( x + 30) = 0

⇒ x = 90, -30

However, the side of the field cannot be negative. Therefore, the length of the shorter side will be 90 m.

And the length of the larger side will be (90 + 30) m = 120 m.

7. The difference of the squares of two numbers is 180. The square of the smaller number is 8 times the larger number. Find the two numbers.

Let us say the larger and smaller number be x and y, respectively.

As per the question given,

x 2 – y 2 = 180 and y 2 = 8 x

⇒ x 2 – 8 x = 180

⇒ x 2 – 8 x – 180 = 0

⇒ x 2 – 18 x + 10 x – 180 = 0

⇒ x ( x – 18) +10( x – 18) = 0

⇒ ( x – 18)( x + 10) = 0

⇒ x = 18, -10

However, the larger number cannot be considered a negative number, as 8 times the larger number will be negative, and hence, the square of the smaller number will be negative, which is not possible.

Therefore, the larger number will be 18 only.

∴ y 2 = 8x = 8 × 18 = 144

⇒ y = ±√144 = ±12

∴ Smaller number = ±12

Therefore, the numbers are 18 and 12 or 18 and -12.

8. A train travels 360 km at a uniform speed. If the speed had been 5 km/h more, it would have taken 1 hour less for the same journey. Find the speed of the train.

It is given that

Distance = 360 km

Consider x as the speed, then the time taken

If the speed is increased by 5 km/h, the speed will be (x + 5) km/h.

Distance will be the same.

t = 360/(x + 5)

We know that

Time with original speed – Time with increased speed = 1

360/x – 360/(x + 5) = 1

LCM = x (x + 5)

360 x + 1800 – 360x = x (x + 5)

x 2 + 5x = 1800

x 2 + 5x – 1800 = 0

x 2 + 45x – 40x – 1800 = 0

x (x + 45) – 40 (x + 45) = 0

(x – 40) (x + 45) = 0

x = 40 km/hr

As we know, the value of speed cannot be negative.

Therefore, the speed of the train is 40 km/h.

NCERT Solutions for Class 10 Chapter 4- 1

Let the time taken by the smaller pipe to fill the tank = x hr.

Time taken by the larger pipe = ( x – 10) hr

Part of the tank filled by smaller pipe in 1 hour = 1/ x

Part of the tank filled by larger pipe in 1 hour = 1/( x – 10)

NCERT Solutions for Class 10 Chapter 4- 2

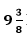
1/ x + 1/ x -10 = 8/75

x -10+ x / x ( x -10) = 8/75

⇒ 2 x -10/ x ( x -10) = 8/75

⇒ 75(2 x – 10) = 8 x 2 – 80 x

⇒ 150 x – 750 = 8 x 2 – 80 x

⇒ 8 x 2 – 230 x +750 = 0

⇒ 8 x 2 – 200 x – 30 x + 750 = 0

⇒ 8 x ( x – 25) -30( x – 25) = 0

⇒ ( x – 25)(8 x -30) = 0

⇒ x = 25, 30/8

Time taken by the smaller pipe cannot be 30/8 = 3.75 hours, as the time taken by the larger pipe will become negative, which is logically not possible.

Therefore, the time taken individually by the smaller pipe and the larger pipe will be 25 and 25 – 10 =15 hours, respectively.

10. An express train takes 1 hour less than a passenger train to travel 132 km between Mysore and Bangalore (without taking into consideration the time they stop at intermediate stations). If the average speed of the express train is 11 km/h more than that of the passenger train, find the average speed of the two trains.

Let us say the average speed of the passenger train = x km/h.

Average speed of express train = ( x + 11) km/h

Given the time taken by the express train to cover 132 km is 1 hour less than the passenger train to cover the same distance. Therefore,

(132/x) – (132/(x+11)) = 1

132(x+11-x)/(x(x+11)) = 1

132 × 11 /(x(x+11)) = 1

⇒ 132 × 11 = x ( x + 11)

⇒ x 2 + 11 x – 1452 = 0

⇒ x 2 + 44 x -33 x -1452 = 0

⇒ x ( x + 44) -33( x + 44) = 0

⇒ ( x + 44)( x – 33) = 0

⇒ x = – 44, 33

As we know, speed cannot be negative.

Therefore, the speed of the passenger train will be 33 km/h and thus, the speed of the express train will be 33 + 11 = 44 km/h.

11. Sum of the areas of two squares is 468 m 2 . If the difference between their perimeters is 24 m, find the sides of the two squares.

Let the sides of the two squares be x m and y m.

Therefore, their perimeter will be 4 x and 4 y, respectively

And the area of the squares will be x 2 and y 2, respectively.

4 x – 4 y = 24

x – y = 6

x = y + 6

Also, x 2 + y 2 = 468

⇒ (6 + y 2 ) + y 2 = 468

⇒ 36 + y 2 + 12 y + y 2 = 468

⇒ 2 y 2 + 12 y + 432 = 0

⇒ y 2 + 6y – 216 = 0

⇒ y 2 + 18 y – 12 y – 216 = 0

⇒ y ( y +18) -12( y + 18) = 0

⇒ ( y + 18)( y – 12) = 0

⇒ y = -18, 12

As we know, the side of a square cannot be negative.

Hence, the sides of the squares are 12 m and (12 + 6) m = 18 m.

Exercise 4.4 Page: 91

1. Find the nature of the roots of the following quadratic equations. If the real roots exist, find them. (i) 2 x 2 – 3 x + 5 = 0 (ii) 3 x 2 – 4√3 x + 4 = 0 (iii) 2 x 2 – 6 x + 3 = 0

2x 2 – 3 x + 5 = 0

Comparing the equation with ax 2 + bx + c = 0, we get

a = 2, b = -3 and c = 5

We know, Discriminant = b 2 – 4 ac

= ( – 3) 2 – 4 (2) (5) = 9 – 40

= – 31

As you can see, b 2 – 4ac < 0

Therefore, no real root is possible for the given equation, 2x 2 – 3 x + 5 = 0

(ii) 3 x 2 – 4√3 x + 4 = 0

a = 3, b = -4√3 and c = 4

= (-4√3) 2 – 4(3)(4)

= 48 – 48 = 0

As b 2 – 4 ac = 0,

Real roots exist for the given equation, and they are equal to each other.

Hence, the roots will be – b /2 a and – b /2 a .

– b /2 a = -(-4√3)/2×3 = 4√3/6 = 2√3/3 = 2/√3

Therefore, the roots are 2/√3 and 2/√3.

(iii) 2 x 2 – 6 x + 3 = 0

a = 2, b = -6, c = 3

As we know, Discriminant = b 2 – 4 ac

= (-6) 2 – 4 (2) (3)

= 36 – 24 = 12

As b 2 – 4 ac > 0,

Therefore, there are distinct real roots that exist for this equation, 2 x 2 – 6 x + 3 = 0

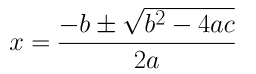
= (-(-6) ± √(-6 2 -4(2)(3)) )/ 2(2)

= (6±2√3 )/4

Therefore, the roots for the given equation are (3+√3)/2 and (3-√3)/2

2. Find the values of k for each of the following quadratic equations so that they have two equal roots. (i) 2 x 2 + kx + 3 = 0 (ii) kx ( x – 2) + 6 = 0

(i) 2 x 2 + kx + 3 = 0

Comparing the given equation with ax 2 + bx + c = 0, we get,

a = 2, b = k and c = 3

= ( k ) 2 – 4(2) (3)

= k 2 – 24

For equal roots, we know,

Discriminant = 0

k 2 – 24 = 0

k = ±√24 = ±2√6

(ii) kx ( x – 2) + 6 = 0

or kx 2 – 2 kx + 6 = 0

Comparing the given equation with ax 2 + bx + c = 0, we get

a = k , b = – 2 k and c = 6

= ( – 2 k ) 2 – 4 ( k ) (6)

= 4 k 2 – 24 k

b 2 – 4 ac = 0

4 k 2 – 24 k = 0

4 k ( k – 6) = 0

Either 4 k = 0 or k = 6 = 0

k = 0 or k = 6

However, if k = 0, then the equation will not have the terms ‘ x 2 ‘ and ‘ x ‘.

Therefore, if this equation has two equal roots, k should be 6 only.

3. Is it possible to design a rectangular mango grove whose length is twice its breadth and the area is 800 m 2 ? If so, find its length and breadth.

Let the breadth of the mango grove be l .

The length of the mango grove will be 2 l .

Area of the mango grove = (2 l ) ( l )= 2 l 2

2 l 2 = 800

l 2 = 800/2 = 400

l 2 – 400 =0

a = 1, b = 0, c = 400

=> (0) 2 – 4 × (1) × ( – 400) = 1600

Here, b 2 – 4 ac > 0

Thus, the equation will have real roots. And hence, the desired rectangular mango grove can be designed.

As we know, the value of length cannot be negative.

Therefore, the breadth of the mango grove = 20 m

Length of mango grove = 2 × 20 = 40 m

4. Is the following situation possible? If so, determine their present ages. The sum of the ages of two friends is 20 years. Four years ago, the product of their age in years was 48.

Let’s say the age of one friend is x years.

Then, the age of the other friend will be (20 – x) years.

Four years ago,

Age of First friend = ( x – 4) years

Age of Second friend = (20 – x – 4) = (16 – x ) years

As per the given question, we can write,

( x – 4) (16 – x ) = 48

16 x – x 2 – 64 + 4 x = 48

– x 2 + 20 x – 112 = 0

x 2 – 20 x + 112 = 0

a = 1 , b = -2 0 and c = 112

Discriminant = b 2 – 4 ac

= (- 20 ) 2 – 4 × 112

= 400 – 448 = -48

b 2 – 4 ac < 0

Therefore, there will be no real solution possible for the equations. Hence, the condition doesn’t exist.

5. Is it possible to design a rectangular park of perimeter 80 and an area of 400 m2? If so, find its length and breadth.

Let the length and breadth of the park be l and b.

Perimeter of the rectangular park = 2 ( l + b ) = 80

So, l + b = 40

Or, b = 40 – l

Area of the rectangular park = l×b = l(40 – l) = 40 l – l 2 = 400

l 2 – 40 l + 400 = 0, which is a quadratic equation.

a = 1, b = -40, c = 400

Since, Discriminant = b 2 – 4 ac

=(- 40 ) 2 – 4 × 400

= 1600 – 1600 = 0

Thus, b 2 – 4 ac = 0

Therefore, this equation has equal real roots. Hence, the situation is possible.

The root of the equation,

l = – b /2 a

l = -(-40)/2(1) = 40/2 = 20

Therefore, the length of the rectangular park, l = 20 m

And the breadth of the park, b = 40 – l = 40 – 20 = 20 m.

NCERT Solutions for Class 10 Maths Chapter 4 – Quadratic Equations

A 1-mark question was asked from Chapter 4 Quadratic Equations in the year 2018. However, in the year 2017, a total of 13 marks were asked from the topic Quadratic Equations. Therefore, students need to have a thorough understanding of the topic. The topics and sub-topics provided in this chapter include:

4.1 Introduction

If we equate the polynomial ax 2 + bx + c, a ≠ 0 to zero, we get a quadratic equation. Quadratic equations come up when we deal with many real-life situations. In this chapter, students will study quadratic equations and various ways of finding their roots. They will also see some applications of quadratic equations in daily life situations.

4.2 Quadratic Equations

A quadratic equation in the variable x is an equation of the form ax 2 + bx + c = 0, where a, b, c are real numbers, a ≠ 0. In fact, any equation of the form p(x) = 0, where p(x) is a polynomial of degree 2, is a quadratic equation. But when we write the terms of p(x) in descending order of their degrees, then we get the standard form of the equation. That is, ax 2 + bx + c = 0, a ≠ 0 is called the standard form of a quadratic equation.

4.3 Solution of Quadratic Equations by Factorisation

A real number α is called a root of the quadratic equation ax 2 + bx + c = 0, a ≠ 0 if a α 2 + bα + c = 0. We also say that x = α is a solution of the quadratic equation or that α satisfies the quadratic equation. Note that the zeroes of the quadratic polynomial ax 2 + bx + c and the roots of the quadratic equation ax 2 + bx + c = 0 are the same.

4.4 Solution of a Quadratic Equation by Completing the Square

Finding the value that makes a quadratic equation a square trinomial is called completing the square. The square trinomial can then be solved easily by factorising.

4.5 Nature of Roots

If b 2 – 4ac < 0, then there is no real number whose square is b 2 – 4ac. Therefore, there are no real roots for the given quadratic equation in this case. Since b 2 – 4ac determines whether the quadratic equation ax 2 + bx + c = 0 has real roots or not, b 2 – 4ac is called the discriminant of this quadratic equation. So, a quadratic equation ax 2 + bx + c = 0 has (i) two distinct real roots, if b 2 – 4ac > 0, (ii) two equal real roots, if b 2 – 4ac = 0, (iii) no real roots, if b 2 – 4ac < 0. 4.6

List of exercises we covered in NCERT Solutions for Class 10 Maths Chapter 4

Exercise 4.1 Solutions – 2 Questions Exercise 4.2 Solutions – 6 Questions Exercise 4.3 Solutions – 11 Questions Exercise 4.4 Solutions – 5 Questions

In a quadratic equation, x represents an unknown form, and a, b, and c are the known values. An equation to be quadratic “a” should not be equal to 0. The equation is of the form ax 2 + bx + c = 0. The values of a, b, and c are always real numbers. A quadratic equation can be calculated by completing the square. A quadratic equation has

Two different real roots
No real roots
Two equal roots

Key Features of NCERT Solutions for Class 10 Maths Chapter 4 – Quadratic Equations

The highly experienced faculty at BYJU’S designs NCERT Solutions with utmost care.
The solutions are 100% accurate and can be used by the students while preparing for their CBSE board exams.
All the minute concepts are also covered to help students face other competitive exams more confidently.
The exercise questions present in the NCERT Textbook have been answered in a step-wise manner so that students attain good scores not only on the final answer but also on each step.

For more questions to practise, students can refer to the other study materials which are given at BYJU’S.

RD Sharma Solutions for Class 10 Maths Chapter 8 Quadratic Equations

Disclaimer –

Dropped Topics – 4.4 Solution of a quadratic equation by completing the squares

Frequently Asked Questions on NCERT Solutions for Class 10 Maths Chapter 4

How many exercises are there in ncert solutions for class 10 maths chapter 4, is byju’s website providing answers for ncert solutions for class 10 maths chapter 4 quadratic equations, mention the important concepts you learn in ncert solutions for class 10 maths chapter 4 quadratic equations., leave a comment cancel reply.

Your Mobile number and Email id will not be published. Required fields are marked *

Request OTP on Voice Call

Post My Comment